1. INTRODUCTION
2. TRANSIENT RESPONSE OF RL CIRCUITS
3. TRANSIENT RESPONSE OF RC CIRCUITS
4. TRANSIENT RESPONSE OF RLC CIRCUITS
5. CHARACTERIZATION OF TWO PORT NETWORKS IN TERMS OF Z,Y AND H PARAMETERS.
1. INTRODUCTION
For higher order differential equation, the number of arbitrary constants equals the order of the equation. If these unknowns are to be evaluated for particular solution, other conditions in network must be known. A set of simultaneous equations must be formed containing general solution and some other equations to match number of unknown with equations.
We assume that at reference time t=0, network condition is changed by switching action. Assume that switch operates in zero time. The network conditions at this instant are called initial conditions in network.
1. Resistor :
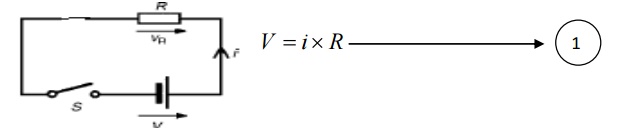
Equ 1 is linear and also time dependent. This indicates that current through resistor changes if applied voltage changes instantaneously. Thus in resistor, change in current is instantaneous as there is no storage of energy in it.
2.Inductor:
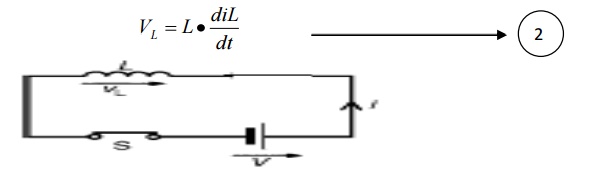
If dc current flows through inductor, dil/dt becomes zero as dc current is constant with respect to time. Hence voltage across inductor, VL becomes zero. Thus, as for as dc quantities are considered, in steady stake, inductor acts as short circuit.
We can express inductor current in terms of voltage developed across it as

I through inductor cannot change instantaneously.
3.capacitor
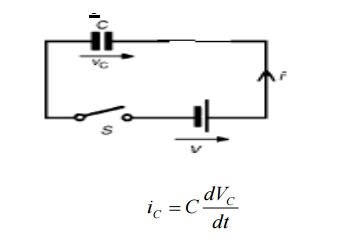
If dc voltage is applied to capacitor, dVC / dt becomes zero as dc voltage is constant with respect to time.
Hence the current through capacitor iC becomes zero, Thus as far as dc quantities are considered capacitor acts as open circuit.
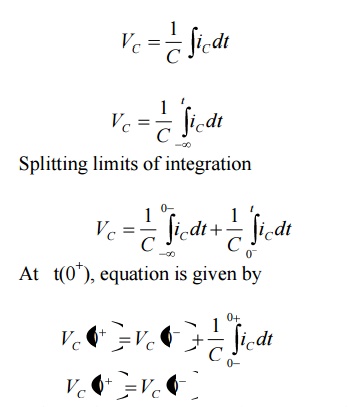
Thus voltage across capacitor can not change instantaneously.
2. TRANSIENT RESPONSE OF RL CIRCUITS:
So far we have considered dc resistive network in which currents and voltages were independent of time. More specifically, Voltage (cause input) and current (effect output) responses displayed simultaneously except for a constant multiplicative factor (VR). Two basic passive elements namely, inductor and capacitor are introduced in the dc network. Automatically, the question will arise whether or not the methods developed in lesson-3 to lesson-8 for resistive circuit analysis are still valid. The voltage/current relationship for these two passive elements are defined by the derivative (voltage across the inductor
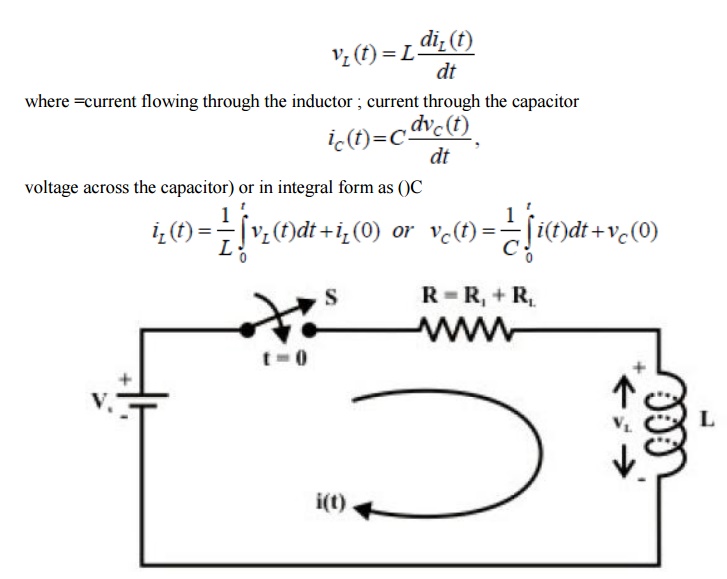
Our problem is to study the growth of current in the circuit through two stages, namely; (i) dc transient response (ii) steady state response of the system
D.C Transients: The behavior of the current and the voltage in the circuit switch is closed until it reaches its final value is called dc transient response of the concerned circuit. The response of a circuit (containing resistances, inductances, capacitors and switches) due to sudden application of voltage or current is called transient response. The most common instance of a transient response in a circuit occurs when a switch is turned on or off –a rather common event in an electric circuit.
Growth or Rise of current in R-L circuit
To find the current expression (response) for the circuit shown in fig. 10.6(a), we can write the KVL equation around the circuit
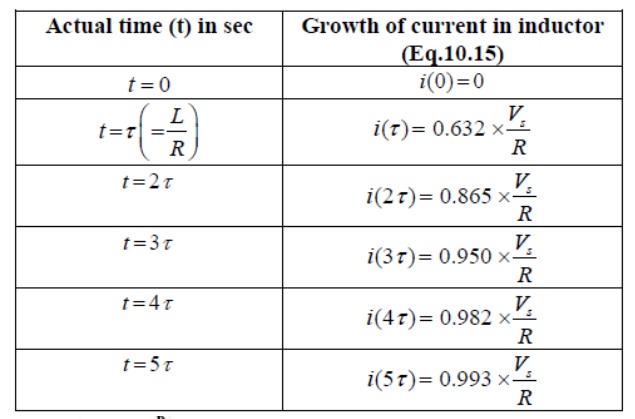
The table shows how the current i(t) builds up in a R-L circuit.
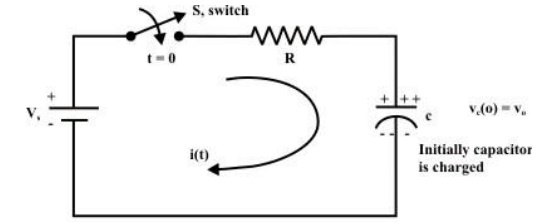
Consider network shown in fig. the switch k is moved from position 1 to 2 at reference time t = 0.
Now before switching take place, the capacitor C is fully charged to V volts and it discharges through resistance R. As time passes, charge and hence voltage across capacitor i.e. Vc decreases gradually and hence discharge current also decreases gradually from maximum to zero exponentially.
After switching has taken place, applying kirchoff’s voltage law,
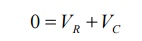
Where VR is voltage across resistor and VC is voltage across capacitor.
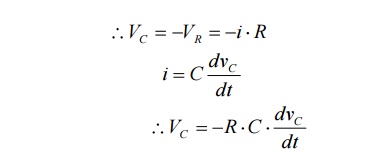
Above equation is linear, homogenous first order differential equation. Hence rearranging we have,

Integrating both sides of above equation we have
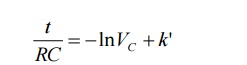
Now at t = 0, VC =V which is initial condition, substituting in equation we have,
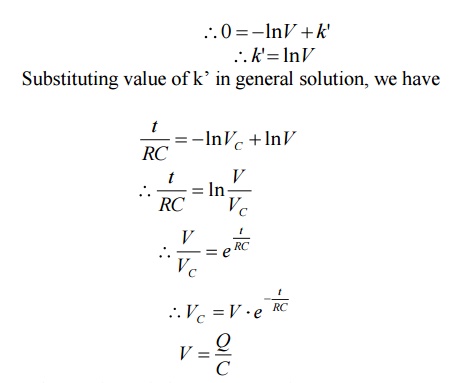
Where Q is total charge on capacitor
Similarly at any instant, VC = q/c where q is instantaneous charge.
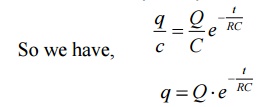
Thus charge behaves similarly to voltage across capacitor.
Now discharging current i is given by
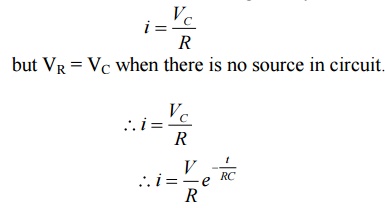
but VR = VC when there is no source in circuit.
The above expression is nothing but discharge current of capacitor. The variation of this current with respect to time is shown in fig.
This shows that the current is exponentially decaying. At point P on the graph. The current value is (0.368) times its maximum value. The characteristics of decay are determined by values R and C, which are 2 parameters of network.
For this network, after the instant t = 0, there is no driving voltage source in circuit, hence it is called undriven RC circuit.

3. TRANSIENT RESPONSE OF RC CIRCUITS
Ideal and real capacitors: An ideal capacitor has an infinite dielectric resistance and plates (made of metals) that have zero resistance. However, an ideal capacitor does not exist as all dielectrics have some
leakage current and all capacitor plates have some resistance. A capacitor’s of how much charge (current) it will allow to leak through the dielectric medium. Ideally, a charged
capacitor is not supposed to allow leaking any current through the dielectric medium and also assumed not to dissipate any power loss in capacitor plates resistance. Under this situation, the model as shown in fig. 10.16(a) represents the ideal capacitor. However, all real or practical capacitor leaks current to some extend due to leakage resistance of dielectric medium. This leakage resistance can be visualized as a resistance connected in parallel with the capacitor and power loss in capacitor plates can be realized with a resistance connected in series with capacitor. The model of a real capacitor is shown in fig.
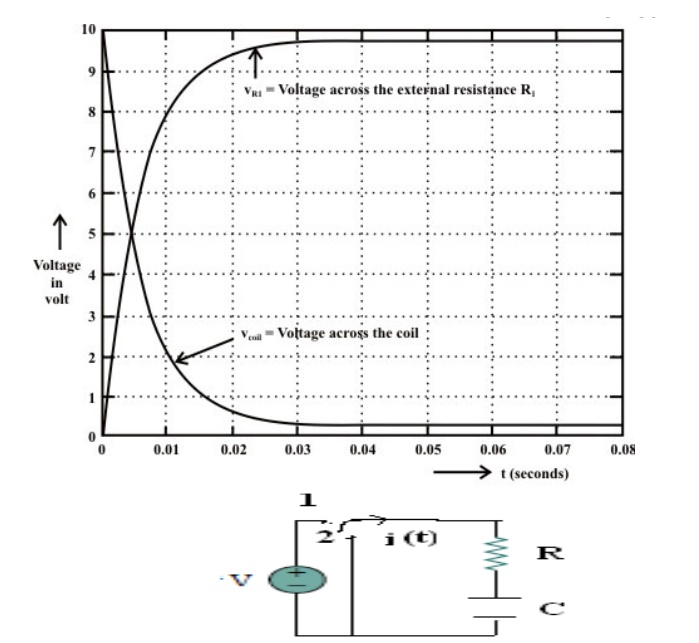
Let us consider a simple series RC−circuit shown in fig. 10.17(a) is connected through a switch ‘S’ to a constant voltage source .
The switch ‘S’ is closed at time ‘t=0’ It is assumed that the capacitor is initially charged with a voltage and the current flowing through the circuit at any instant of time ‘’ after closing the switch is
Current decay in source free series RL circuit: -
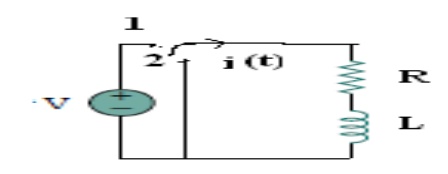
At t = 0- , , switch k is kept at position ‘a’ for very long time. Thus, the network is in steady state. Initial current through inductor is given as,
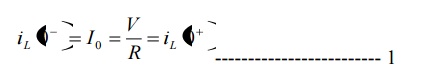
Because current through inductor can not change instantaneously
Assume that at t = 0 switch k is moved to position 'b'.
Applying KVL,
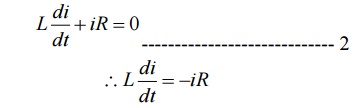
Rearranging the terms in above equation by separating variables
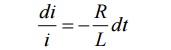
Integrating both sides with respect to corresponding variables

Where k’ is constant of integration.
To find- k’:
Form equation 1, at t=0, i=I0
Substituting the values in equation 3
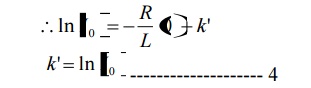
Substituting value of k’ from equation 4 in
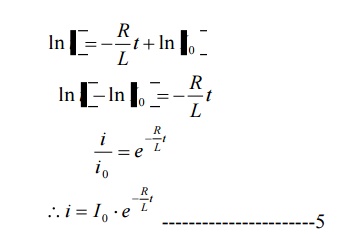
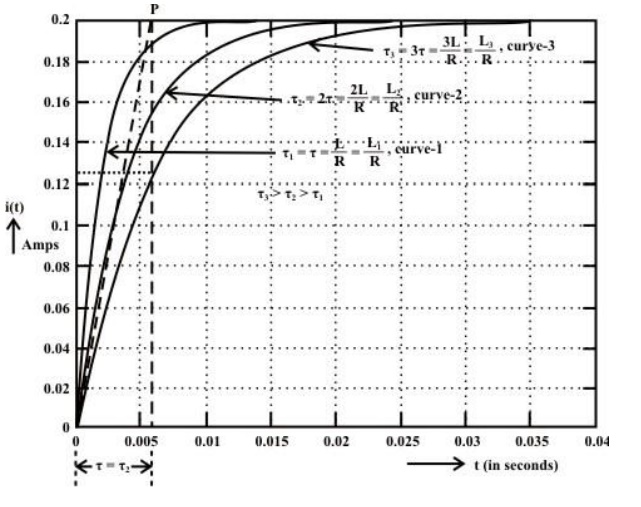
fig. shows variation of current i with respect to time
From the graph, H is clear that current is exponentially decaying. At point P on graph. The current value is (0.363) times its maximum value. The characteristics of decay are determined by values R and L which are two parameters of network.
The voltage across inductor is given by
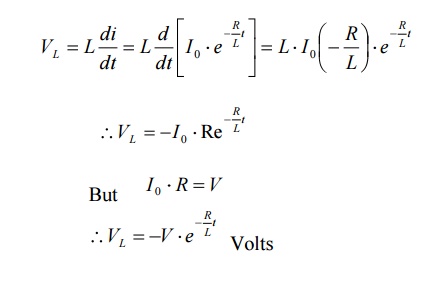
4. TRANSIENT RESPONSE OF RLC CIRCUITS
In the preceding lesson, our discussion focused extensively on dc circuits having resistances with either inductor () or capacitor () (i.e., single storage element) but not both. Dynamic response of such first order system has been studied and discussed in detail. The presence of resistance, inductance, and capacitance in the dc circuit introduces at least a second order differential equation or by two simultaneous coupled linear first order differential equations. We shall see in next section that the complexity of analysis of second order circuits increases significantly when compared with that encountered with first order circuits. Initial conditions for the circuit variables and their derivatives play an important role and this is very crucial to analyze a second order dynamic system.
Response of a series R-L-C circuit
Consider a series RLcircuit as shown in fig.11.1, and it is excited with a dc voltage source C−−sV.
Applying around the closed path for ,
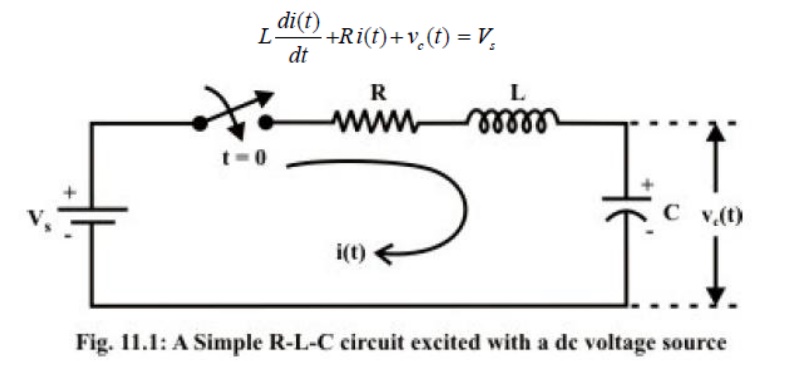
The current through the capacitor can be written as Substituting the current ‘’expression in eq.(11.1) and rearranging the terms,
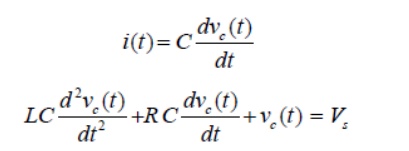
The above equation is a 2nd-order linear differential equation and the parameters associated with the differential equation are constant with time. The complete solution of the above differential equation has two components; the transient response and the steady state response. Mathematically, one can write the complete solution as
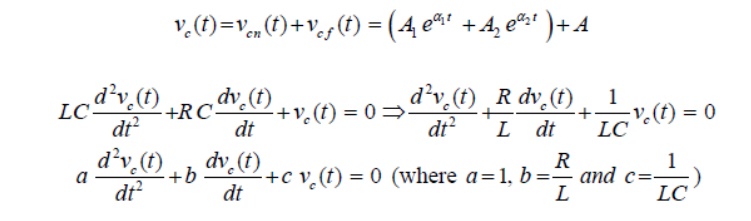
Since the system is linear, the nature of steady state response is same as that of forcing function (input voltage) and it is given by a constant value. Now, the first part of the total response is completely dies out with time while and it is defined as a transient or natural response of the system. The natural or transient response (see Appendix in Lesson-10) of second order differential equation can be obtained from the homogeneous equation (i.e., from force free system) that is expressed by

and solving the roots of this equation (11.5) on that associated with transient part of the complete solution (eq.11.3) and they are given below.

The roots of the characteristic equation are classified in three groups depending upon the values of the parameters ,,RLand of the circuit
Case-A (overdamped response): That the roots are distinct with negative real parts. Under this situation, the natural or transient part of the complete solution is written as

and each term of the above expression decays exponentially and ultimately reduces to zero as and it is termed as overdamped response of input free system. A system that is overdamped responds slowly to any change in excitation. It may be noted that the exponential term t→∞11tAeαtakes longer time to decay its value to zero than the term21tAeα. One can introduce a factorξ that provides an information about the speed of system response and it is defined by damping ratio
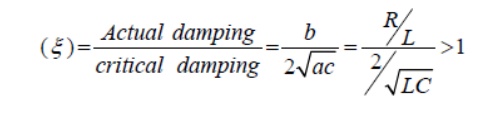
RLC Circuit:
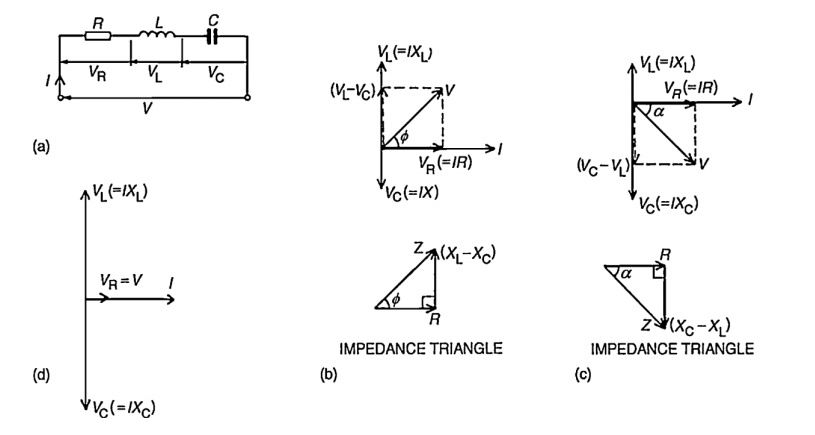
Consider a circuit in which R, L, and C are connected in series with each other across ac supply as shown in fig.
The ac supply is given by, V = Vm sin wt
The circuit draws a current I. Due to that different voltage drops are,
1. Voltage drop across Resistance R is VR = IR
2. Voltage drop across Inductance L is VL = IXL
3. Voltage drop across Capacitance C is Vc = IXc The characteristics of three drops are,
(i) VR is in phase with current I
(ii) VL leads I by 900
(iii) Vc lags I by 900
According to krichoff’s laws
Steps to draw phasor diagram:
1. Take current I as reference
2. VR is in phase with current I
3. VL leads current by 900
4. Vc lags current by 900
5. obtain resultant of VL and Vc. Both VL and Vc are in phase opposition (1800 out of phase)
6. Add that with VRby law of parallelogram to get supply voltage.
The phasor diagram depends on the condition of magnitude of VL and Vc which ultimately depends on values of XL and Xc.
Let us consider different cases:
Case(i): XL > Xc
When X L > Xc
Also VL > Vc (or) IXL > IXc
So, resultant of VL and Vc will directed towards VL i.e. leading current I. Hence I lags V i.e. current I will lags the resultant of VL and Vc i.e. (V L - Vc). The circuit is said to be inductive in nature.
From voltage triangle,
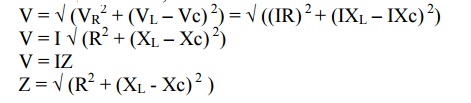
If , V = Vm Sin wt ; i = Im Sin (wt - ф )
i.e I lags V by angle ф
Case(ii): XL < Xc
When XL < Xc
Also VL < Vc (or) IXL < IXc
Hence the resultant of VL and Vc will directed towards Vc i.e current is said to be capacitive in nature
Form voltage triangle
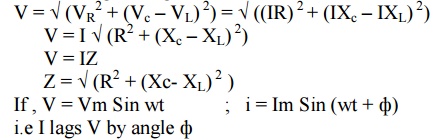
i.e I lags V by angle ф
Case(iii): XL = Xc
When XL = Xc
Also VL = Vc (or) IXL = IXc
So VL and Vc cancel each other and the resultant is zero. So V = VR in such a case, the circuit is purely resistive in nature.

