The torque-speed characteristics shown in Fig.2.4b are drawn on the crude assumption that the armature current is continuous over the whole range of operation. It is very doubtful that the armature current will be continuous at high values of the firing angle a, high speed, and low values of torque. In fact, armature current is discontinuous for these operating conditions. If the armature current is discontinuous, the no-load speeds will be higher than those shown in Fig.2.4b, and the speed regulation will be significantly poor in the region of discontinuous armature current. The motor performance
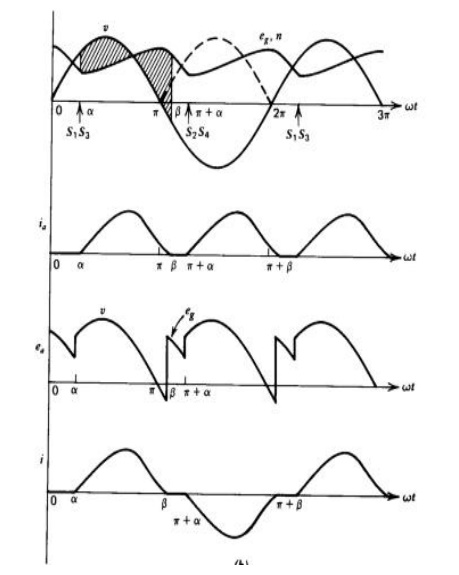
In Fig. 2.5, the motor is connected to the input supply for the period a<wI <71' through S, and Dz.Beyond 71', the motor terminal is shorted through the free-wheeling diode DFW' The armature current decays to zero at before the thyristor S2 is triggered at71' +a, thereby making the armature current discontinuous. During a to 71' (i.e., the conduction period of the thyristor S,), motor terminal voltage ea is the same as the supply voltage v. However, during the motor current free-wheels through DFW and so ea s zero. The motor coasts and the motor terminal voltage ea is the same as the back voltage InFig.2.6, the motor is connected to the supply during a<wt<{3 and it Coasts during {3<wI <71' +a. As long as the motor is connected to the supply, its terminal voltage is the same as the input supply voltage.
If the armature current can be assumed to be continuous, the torque-speed characteristics can be calculated merely from average values ofthe motor terminal voltage and current. In the discontinuous current mode, these calculations are cumbersome. The difficulty arises in the calculation of the average motor terminal voltage Ea, because (called the extinction angle, the instant at which the thyristor or motor current becomes zero) depends on, the average speed N, average armature current la' and the firing angle a. A general approach, valid for both continuous and discontinuous armature current, is therefore necessary.

